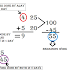
LCM and HCF

LCM and HCF of Fractions

• LCM of fractions = (LCM of numerators)/(HCF of denominators)

• HCF of fractions = (HCF of numerators)/(LCM of denomenators)

Solution Methods:

• Product of two numbers = HCF of the numbers * LCM of the numbers.

• The greatest number which divides the numbers x, y and z, leaving remainders a, b and c respectively is given by

HCF of (x-a), (y-b), (z-c)

• The least number which when divided by x, y and z leaves the remainders a, b and c respectively is given by

LCM of (x, y, z) - k

where,   K = (x-a) = (y-b) = (z-c)

• The least number which when divided by x, y and z leaves the same remainder k in each case, is given by

LCM of (x, y, z) + k

• The greatest number that will divide x, y and z leaving the same remainder in each case is given by

HCF of (x-y), (y-z), (z-x)

• The greatest n - digit number which when divided by x, y and z:

      (a) Leaves no remainder

Requires number = n-digit greatest number - R

      (b) Leaves remainder k

 Required number = [n-digit greatest number - R] + k

where R is the remainder obtained when n-digit greatest number is divided by the LCM of x, y and z.

• The smallest n-digit number which when divided by x, y ans z leaves

      (a) No remainder

Required number = [n-digit smallest number  + (L-R)]

     (b) Remainder k

Required number = [n-digit smallest number + (L-R)] + k

where, R is the number obtained when n-digit smallest number is divided by LCM of x, y, and z. L is the LCM of x, y and z.